


Next: 5.4 Sensitivity of the
Up: 5. Detection and Observation
Previous: 5.2 Detection and Observation
Contents
5.3 Statistical Formulae
The problem of the detection/observation of an astronomical object
superposed on a smooth sky background and imaged with a CCD detector can be
mathematically described as the general problem of the detection/observation
of a Poisson signal (photoelectrons originating from the object under
consideration) in presence of Poisson noise (photoelectrons originating from
the sky background) and Gaussian noise (readnoise).
Following a simple statistical approach, general analytical formulae for the
signal-to-noise ratios and standard errors of CCD photometry can be derived,
which are convenient to use during the instrument design and mission planning
phases because they give very similar errors to those obtained from
simulations.
The use of analytical formulae has the additional advantage over simulations
that the relative importance of the various error sources is more easily seen.
Our goal is to derive general formulae expressing the signal-to-noise ratio
produced by an object within a given aperture during a given exposure time,
and then to apply them to the cases of detection and observation.
As for detection, the estimation of the signal-to-noise ratio leads to
estimate the number of galaxies that could be detected under the present
assumptions for sampling and readnoise in the ASM1.
As for observation, a comparison between the estimated accuracies
in surface photometry achievable with different sample sizes can be given.
The conventions used in the following are as follows:
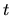 : single-scan exposure time [s]
: single-scan exposure time [s]
- aperture : the angular size of the sky area over which the measurement is
performed [solid angle]
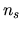 : number of samples within the aperture
: number of samples within the aperture
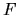 : total electron counts within the aperture during
: total electron counts within the aperture during 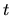 [e
[e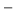 ]
]
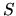 : electron counts from the object within the aperture during
: electron counts from the object within the aperture during 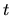 [e
[e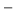 ]
]
 : electron counts from the sky background per sample during
: electron counts from the sky background per sample during 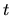 [e
[e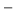 ]
]
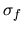 : standard error of generic estimated flux
: standard error of generic estimated flux 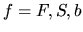 [e
[e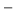 ]
]
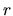 : total readnoise per sample [e
: total readnoise per sample [e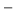 rms]
rms]
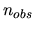 : number of superposed observations
: number of superposed observations
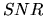 : signal-to-noise ratio in the measurement of
: signal-to-noise ratio in the measurement of 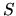
-
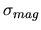 : relative standard error in the measurement of
: relative standard error in the measurement of 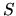 [mag]
[mag]
Under these assumptions, 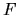 can be written as
can be written as
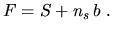 |
(5.1) |
The physical process of the emission of photons fron an astronomical object
can be statistically described in terms of a Poisson distribution.
The standard error in the measurement of 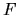 is then due to the intrinsic
Poisson noise associated with
is then due to the intrinsic
Poisson noise associated with 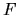 and to the readnoise.
These two contributions sum quadratically yielding for the variance of
and to the readnoise.
These two contributions sum quadratically yielding for the variance of 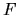
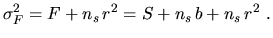 |
(5.2) |
Since the signal 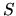 from the object is estimated by subtraction from
from the object is estimated by subtraction from 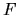 of
the sky background
of
the sky background
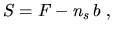 |
(5.3) |
the variance of 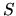 is
is
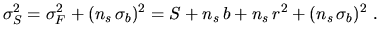 |
(5.4) |
 is then
is then
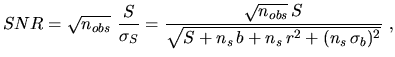 |
(5.5) |
while
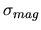 is
is
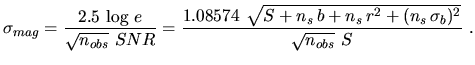 |
(5.6) |
Even though 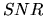 is given by Equation 5.5 both for detection and for
observation, its meaning is very different in the two cases.
In the case of detection, an high
is given by Equation 5.5 both for detection and for
observation, its meaning is very different in the two cases.
In the case of detection, an high 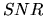 means a safe detection, i.e. an high
chance of having detected a galaxy.
As for observation, an high
means a safe detection, i.e. an high
chance of having detected a galaxy.
As for observation, an high 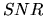 means an accurate observation, i.e. a small error in surface photometry.
In order to apply the previous formulae, one needs to estimate the sensitivity,
the readnoise and the sky background levels of the Astros.
means an accurate observation, i.e. a small error in surface photometry.
In order to apply the previous formulae, one needs to estimate the sensitivity,
the readnoise and the sky background levels of the Astros.



Next: 5.4 Sensitivity of the
Up: 5. Detection and Observation
Previous: 5.2 Detection and Observation
Contents
Mattia Vaccari
2000-12-05